17 18 As A Decimal

Wondering how to convert decimals to fractions? Or how to convert fractions to decimals? It'due south easier than you recollect! Proceed reading to see the steps for decimal to fraction conversions (including why you need to follow different steps if you take a repeating decimal), steps for fraction to decimal conversions, a handy chart with common decimal/fraction conversions, and tips for quickly estimating conversions.

How to Catechumen Decimals to Fractions

How do you catechumen a decimal to a fraction? Whatever decimal, even complicated-looking ones, can be converted to a fraction; you just demand to follow a few steps. Beneath we explain how to convert both terminating decimals and repeating decimals to fractions.

Converting a Terminating Decimal to a Fraction

A terminating decimal is any decimal that has a finite other of digits. In other words, it has an end. Examples include .five, .234, .864721, etc. Terminating decimals are the well-nigh common decimals you'll run across and, fortunately, they are also the easiest to convert to fractions.

Step ane

Write the decimal divided by one.

For case, say you're given the decimal .55.Your first step is to write out the decimal and so information technology looks like ${.55}/{1}$.

Step 2

Next, you lot want to multiply both the top and bottom of your new fraction by x for every digit to the left of the decimal bespeak.

In our instance, .55 has two digits after the decimal indicate, then we'll want to multiply the entire fraction by 10 x 10, or 100. Multiplying the fraction by ${100}/{100}$ gives us ${55}/{100}$.

Step iii

The final step is reducing the fraction to its simplest form. The simplest form of the fraction is when the peak and bottom of the fraction are the smallest whole numbers they tin can exist. For example, the fraction ${3}/{nine}$ isn't in its simplest course considering information technology tin can all the same be reduced down to ⅓ by dividing both the top and bottom of the fraction by 3.

The fraction ${55}/{100}$ can be reduced by dividing both the top and bottom of the fraction by 5, giving the states ${11}/{20}$. xi is a prime number and can't be divided any more, so we know this is the fraction in its simplest class.

The decimal .55 is equal to the fraction ${eleven}/{20}$.

Instance

Convert .108 to a fraction.

After putting the decimal over 1, we end up with ${.108}/{ane}$.

Since .108 has three digits subsequently the decimal identify, we demand to multiply the entire fraction by 10 x 10 x 10, or 1000. This gives us ${108}/{1000}$.

Now we need to simplify. Since 108 and k are both even numbers, we know nosotros can split both by 2. This gives united states ${54}/{500}$. These are still even numbers, then we tin can split past 2 once again to get ${27}/{250}$. 27 isn't a factor of 250, and so the fraction can't be reduced whatever more.

The last answer is ${27}/{250}$.

Converting a Repeating Decimal to a Fraction

A repeating decimal is one that has no end. Since y'all tin't go on writing or typing the decimal out forever, they are oftentimes written as a string of digits rounded off (.666666667) or with a bar above the repeating digit(s) $\ov {(.half dozen)}$.

For our instance, we'll convert .6667 to a fraction.

The decimal .6667 is equal to $\ov {(.half dozen)}$, .666666667, .667, etc. They're all only different ways to testify that the decimal is really a string of 6'southward that goes on forever.

Stride 1

Let 10 equal the repeating decimal you're trying to convert, and identify the repeating digit(due south).

So x=.6667

6 is the repeating digit, and the stop of the decimal has been rounded up.

Step ii

Multiply by whatever value of 10 you demand to get the repeating digit(s) on the left side of the decimal.

For .6667, nosotros know that 6 is the repeating digit. We want that six on the left side of the decimal, which ways moving the decimal place over i spot. So we multiply both sides of the equation by (x ten 1) or 10.

10x = half-dozen.667

Note: You only desire one "set" of repeating digit(s) on the left side of the decimal. In this example, with 6 as the repeating digit, yous only desire one 6 on the left of the decimal. If the decimal was 0.58585858, you'd but want one set of "58" on the left side. If it helps, y'all can picture all repeating decimals with the infinity bar over them, so .6667 would be$\ov {(.vi)}$.

Step iii

Next nosotros want to get an equation where the repeating digit is just to the correct of the decimal.

Looking at x = .6667, we can encounter that the repeating digit (6) is already just to the right of the decimal, so nosotros don't need to do any multiplication. Nosotros'll keep this equation as x = .6667

Step iv

Now we need to solve for x using our two equations, x = .667 and 10x = six.667.

10x - x =6.667-.667

9x = 6

x = ${6}/{9}$

x = ⅔

Example

Convert 1.0363636 to a fraction.

This question is a bit trickier, but we'll be doing the same steps that we did above.

First, make the decimal equal to x, and make up one's mind the repeating digit(south). x = 1.0363636 and the repeating digits are 3 and 6

Next, become the repeating digits on the left side of the decimal (over again, you simply desire one set of repeating digits on the left). This involves moving the decimal iii places to the correct, so both sides need to be multiplied by (10 x 3) or 1000.

1000x = 1036.363636

Now get the repeating digits to the correct of the decimal. Looking at the equation x = 1.0363636, y'all tin can see that there currently is a zero between the decimal and the repeating digits. The decimal needs to be moved over 1 space, so both sides need to be multiplied past ten ten 1.

10x = ten.363636

At present use the two equations, 1000x = 1036.363636 and 10x = x.363636, to solve for ten.

1000x - 10x = 1036.363636 - 10.363636

990x = 1026

10 = ${1026}/{990}$

Since the numerator is larger than the denominator, this is known every bit an irregular fraction. Sometimes you can leave the fraction as an irregular fraction, or you may exist asked to convert it to a regular fraction. You can do this past subtracting 990/990 from the fraction and making it a 1 that'll go adjacent to the fraction.

${1026}/{990}$ - ${990}/{990}$ = 1 ${36}/{990}$

x = 1 ${36}/{990}$

${36}/{990}$ can be simplified by dividing it past xviii.

x = 1 ${ii}/{55}$

How to Convert Fractions to Decimals

The easiest way to convert a fraction to a decimal is but to employ your reckoner. The line betwixt the numerator and denominator acts as a sectionalisation line, and then ${7}/{29}$ equals 7 divided by 29 or .241.

If yous don't have access to a calculator though, you can all the same convert fractions to decimals past using long sectionalisation or getting the denominator to equal a multiple of ten. Nosotros explain both these methods in this section.

Long Segmentation Method

Convert ${3}/{8}$ to a decimal.

Here is what ${3}/{8}$ looks like worked out with long segmentation.

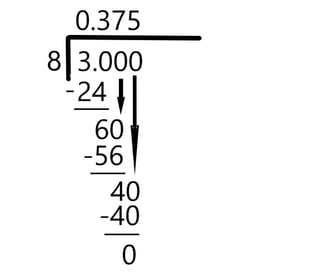
⅜ converted to a decimal is .375

Denominator every bit a Value of x Method

Convert ${3}/{8}$ to a decimal.

Step 1

We want the denominator, in this instance 8, to equal a value of ten. We can do this by multiplying the fraction by 125, giving us ${375}/{one thousand}$.

Step two

Next we want to get the denominator to equal 1 so we can get rid of the fraction. We'll do this by dividing each part of the fraction past thousand, which means moving the decimal over iii places to the left.

This gives us ${.375}/{ane}$ or just .375, which is our answer.

Annotation that this method but works for a fraction with a denominator that can hands be multiplied to be a value of ten. However, there is a trick you tin can utilize to estimate the value of fractions yous can't convert using this method. Check out the case beneath.

Example

Catechumen ⅔ to a decimal.

There is no number you can multiply iii past to arrive an verbal multiple of 10, but you tin can become close.

Past multiplying ⅔ past ${333}/{333}$, nosotros get ${666}/{999}$.

999 is very close to thousand, so let's human action like it actually is 1000, split each role of the fraction past grand, and move the decimal identify of 666 three places to the left, giving us .666

The exact decimal conversion of ⅔ is the repeating decimal .6666667, only .666 gets us very shut.

Then whenever you have a fraction whose denominator can't easily be multiplied to a value of 10 (this will happen to all fractions that convert to repeating decimals), but get the denominator as close to a multiple of 10 as possible for a shut guess.

Common Decimal to Fraction Conversions

Below is a chart with common decimal to fraction conversions. You don't need to memorize these, but knowing at least some of them off the elevation of your caput will make information technology easy to practise some mutual conversions. If you're trying to convert a decimal or fraction and don't have a calculator, you lot can as well see which value in this chart the number is closest to then y'all can brand an educated estimate of the conversion.

Decimal	Fraction
0.03125	${1}/{32}$
0.0625	${1}/{16}$
0.ane	${ane}/{x}$
0.1111	${ane}/{9}$
0.125	${1}/{8}$
0.16667	${1}/{6}$
0.two	${1}/{5}$
0.2222	${2}/{9}$
0.25	${one}/{4}$
0.3	${3}/{10}$
0.3333	${i}/{iii}$
0.375	${iii}/{eight}$
0.4	${2}/{five}$
0.4444	${four}/{9}$
0.5	${1}/{ii}$
0.5555	${v}/{nine}$
0.vi	${three}/{v}$
0.625	${5}/{8}$
0.6666	${two}/{3}$
0.7	${seven}/{10}$
0.75	${3}/{4}$
0.7777	${seven}/{9}$
0.eight	${4}/{five}$
0.8333	${5}/{6}$
0.875	${7}/{8}$
0.8888	${8}/{nine}$
0.9	${9}/{10}$

Summary: How to Make a Decimal Into a Fraction

If you're trying to convert a decimal to fraction, first you demand to decide if it's a terminal decimal (one with an end) or a repeating decimal (i with a digit or digit that repeats to infinity). Once you've done that, you tin follow a few steps for the decimal to fraction conversion and for writing decimals every bit fractions.

If you're trying to catechumen a fraction to decimal, the easiest fashion is just to employ your computer. If you don't have one handy, you can apply long division or get the denominator equal to a multiple of ten, then move the decimal place of the numerator over.

For quick estimates of decimal to fraction conversions (or vice versa), you can wait at our chart of mutual conversions and run across which is closest to your figure to get a ballpark idea of its conversion value.

What's Next?

Want to know the fastest and easiest ways to convert between Fahrenheit and Celsius? We've got you covered! Bank check out our guide to the all-time ways to convert Celsius to Fahrenheit (or vice versa).

Are you learning about logarithms and natural logs in math class?We take a guide on all the natural log rules you need to know.

Did y'all know that water has a very special density? Bank check out our guide to acquire what the density of water is and how the density can change.

Need more help with this topic? Check out Tutorbase!

Our vetted tutor database includes a range of experienced educators who can help you smoothen an essay for English or explain how derivatives piece of work for Calculus. Y'all can use dozens of filters and search criteria to find the perfect person for your needs.

Accept friends who as well need assistance with test prep? Share this article!

About the Author

Christine graduated from Michigan State University with degrees in Environmental Biology and Geography and received her Master's from Duke University. In high school she scored in the 99th percentile on the SAT and was named a National Merit Finalist. She has taught English and biology in several countries.

17 18 As A Decimal,

Source: https://blog.prepscholar.com/convert-decimal-to-fraction

Posted by: howarddiseve.blogspot.com

Share this post